Applicant
Prof. Dr. Ulrich Rüde
Prof. Dr. Harald Köstler
Chair for Computer Science 10 – System simulation
Friedrich-Alexander-Universität Erlangen-Nürnberg
Project Summary
waLBerla is a modern Open-Source software framework whose focus is on the lattice Boltzmann method (LBM) as an alternative to classical Navier-Stokes solvers for computational fluid dynamics, but it can be used to efficiently implement many structured-grid based algorithms.
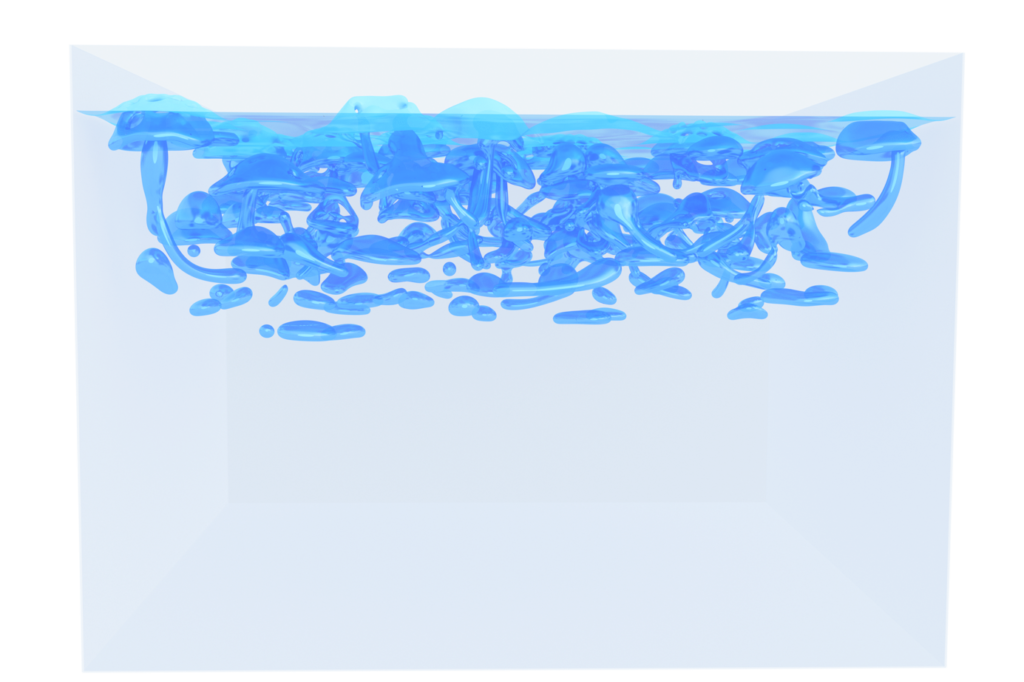
During this project, we developed a high-performance implementation of a multiphase lattice Boltzmann method based on the conservative Allen-Cahn model supporting high-density ratios and high Reynolds numbers. Code generation techniques were used to obtain optimized code for CPUs and GPUs automatically. The coupled model was specified in a high-level symbolic description and optimized through automatic transformations. The memory footprint of the resulting algorithm was reduced through the fusion of compute kernels. A roofline analysis demonstrated the excellent efficiency of the generated code on a single GPU. The resulting single GPU code has been integrated into waLBerla to run massively parallel simulations on large domains. Communication hiding and GPUDirect-enabled MPI yielded near-perfect scaling behaviour. Scaling experiments were conducted with up to 2048 GPUs, simulating several hundred fully resolved bubbles. Further, validation of the implementation was shown in a physically relevant scenario – a three-dimensional rising air bubble in water (cf. figure).
Resulting Publications during KONWIHR project:
Holzer, M., Bauer, M., Köstler, H., & Rüde, U. (2021). Highly efficient lattice Boltzmann multiphase simulations of immiscible fluids at high-density ratios on CPUs and GPUs through code generation. The International Journal of High Performance Computing Applications, 35(4), 413-427.
Mitchell, T., Holzer, M., Schwarzmeier, C., Bauer, M., Rüde, U., & Leonardi, C. (2021). Stability assessment of the phase-field lattice Boltzmann model and its application to Taylor bubbles in annular piping geometries. Physics of Fluids, 33(8), 083325.

